Thickness and Height Pattern
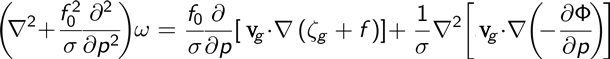
Exercises
Exercise 1
Set the widget to the northern hemisphere and a zonal thickness pattern. Turn on the 500hPa heights, 500-1000hPa thickness and 1000hPa heights. Answer each question below by clicking "done" to see answers and subsequent questions.
Question 1 of 6
Turn on the interrogation points at upper right under “basics”. What is the 500hPa height value over the center of one of the 1000hPa highs? Select the correct answer from the following:
5720 m is the value of the 500 hPa height directly over the center of the 1000 hPa high. This question was to make sure you are correctly interpreting which contours are which and to show you how the interrogation points work.
Question 2 of 6
Now, leave the variables from the previous question on, and turn on the 500hPa winds. Where would you expect the greatest relative vorticity to exist? Choose the best answer.)
The relative vorticity is positive within the trough and strongest near the greatest curvature and cyclonic shear. Turn on the 500 hPa relative vorticity to confirm.
Question 3 of 6
Next, turn off 500 hPa relative vorticity and turn on 500hPa differential absolute vorticity advection. Where is this variable positive with respect to the 500hPa height pattern? (Choose the best answer.)
The 500 hPa differential vorticity advection is maximized downstream, or to the east of, the 500 hPa trough axis, and it extends until the ridge axis - everywhere that the wind vectors cross from a higher value of relative vorticity toward a lower one.
Question 4 of 6
Next, turn off the 500hPa differential vorticity advection and turn on 1000-500hPa thickness advection under “RHS Term 2”. How does the location of the positive thickness advection compare to the location of positive differential absolute vorticity advection? Choose the best answer.
They occur in the same place!
Question 5 of 6
Briefly explain why thickness advection is positive in this region.
(Type your answer in the box, then click Done.)
Thickness lines are zonal, and thickness increases to the south. Thus, any winds that have a southerly component will be advecting higher values of thickness toward lower values. Winds all have some southerly contribution to the east of the trough axis. Thickness advection is strongest in the center of the jet due to faster winds there.
Question 6 of 6
Given this information, what sign should 500hPa omega (the left-hand side of the equation) be to the east of the trough axis?
Omega will be negative and minimized to the east of the trough. Recall that omega is negative for upward motion because of its dependence on pressure instead of height, and that the LHS differential operators behave qualitatively like a negative sign for sinusoidal patterns. Since both differential vorticity advection and thickness advection, the two main variables on the right-hand side, are positive and centered on the jet axis east of the trough, omega should be strong and negative there, indicating ascent. To confirm, turn on "500 hPa Omega" under the LHS overlays.
The question series above concludes exercise 1. Hopefully you are now somewhat familiar with plotting each variable and have an understanding for some of the dynamics of the basic zonal thickness case. Explore the variables in the right-hand side and for 500hPa omega itself for the -pi/2, 0, and pi/2 thickness pattern cases, and then answer the questions in exercises 2 and/or 3 - be sure to consult your instructor to see which exercises are assigned and whether you need to write your answers up to hand in. You may want to jot down some notes about what you see during each case, paying special attention to the distribution/placement of areas of positive and negative differential absolute vorticity advection and thickness advection.
Exercise 2
After examining the -pi/2, 0 and pi/2 widget cases (and revisiting the zonal case if so inclined), answer the questions below. Consult your instructor to determine which exercises are required and in what format they should be completed.
- Which phase shift resulted in the strongest differential absolute vorticity advection, and why?
- Which phase shift(s) resulted in 1000-500mb thickness advection maxima and minima being offset either latitudinally, longitudinally, or both, from the 500mb trough/ridge axes? Why? Explain in terms of thickness gradient and geostrophic wind.
- Do the differential absolute vorticity advection term and thickness advection term always contribute to overall value of omega in the same manner (e.g. both contribute positively in the same location), or do they sometimes counteract each other? If they counteract, explain an example in which this occurred during your exploration.
- Which phase shift shows a situation in which the low pressure system would weaken? Explain why.
- Which phase shift shows a situation in which the low pressure system would strengthen? Explain why./li>
Exercise 3
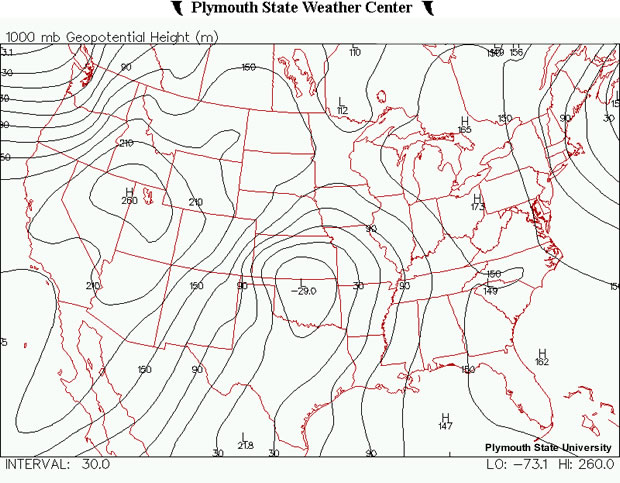
After examining the -pi/2, 0 and pi/2 cases and answering the questions in Exercise 2, apply your knowledge to answer the questions using the imagery below. The first image contains contours of 1000mb heights, and the second image contains black contours of 500mb heights overlaid on color-shaded 1000-500mb thickness. Consult your instructor to determine which exercises are required and in what format they should be completed.
1. Which phase change does this situation most closely represent? (-pi/2, 0 or pi/2)
2. Approximately where would you expect differential geostrophic absolute vorticity advection to be maximized according to the 500mb height map? Minimized? You can use parts of states, cities or landmarks to narrow your description.
3. Approximately where would you expect geostrophic thickness advection to be maximized? Minimized? You can use parts of states, cities or landmarks to narrow your description.
4. Summarize how and where the two terms would combine to create total ascent (negative omega values).
5. Through experience, you know that the precipitation patterns within a midlatitude wave train do not exactly match the regions of ascent that we've outlined in this example and within the widget. What kinds of processes are missing from the QG Omega Equation that help account for the difference in the omega field and the cloud/precipitation shields?